A recap of the some of the biggest scientific breakthroughs over the past two years.Read More
A recap of the some of the biggest scientific breakthroughs over the past two years.Read More
Our AI Opportunity Initiative comes to the Middle East and North Africa
 Google is committed to make benefits of AI more accessible and inclusive for everyone in the Middle East and North Africa.Read More
Google is committed to make benefits of AI more accessible and inclusive for everyone in the Middle East and North Africa.Read More
How we’re helping partners with improved and expanded AI-based flood forecasting
 We’re expanding flood forecasting to over 100 countries and making our breakthrough AI model available to researchers and partners.Read More
We’re expanding flood forecasting to over 100 countries and making our breakthrough AI model available to researchers and partners.Read More
7 pieces of AI news we announced in October
 Here are 7 of Google’s latest AI updates from October.Read More
Here are 7 of Google’s latest AI updates from October.Read More
4 experiments with voice AI models to help you explore culture
 Here are four AI voice models from Google Arts & Culture that offer a new way to experience and engage with art, history and culture.Read More
Here are four AI voice models from Google Arts & Culture that offer a new way to experience and engage with art, history and culture.Read More
Ask a Techspert: What’s the difference between a CPU, GPU and TPU?
 Learn more from a Google expert about CPUs, GPUs and TPUs — and Google latest TPU, Trillium.Read More
Learn more from a Google expert about CPUs, GPUs and TPUs — and Google latest TPU, Trillium.Read More
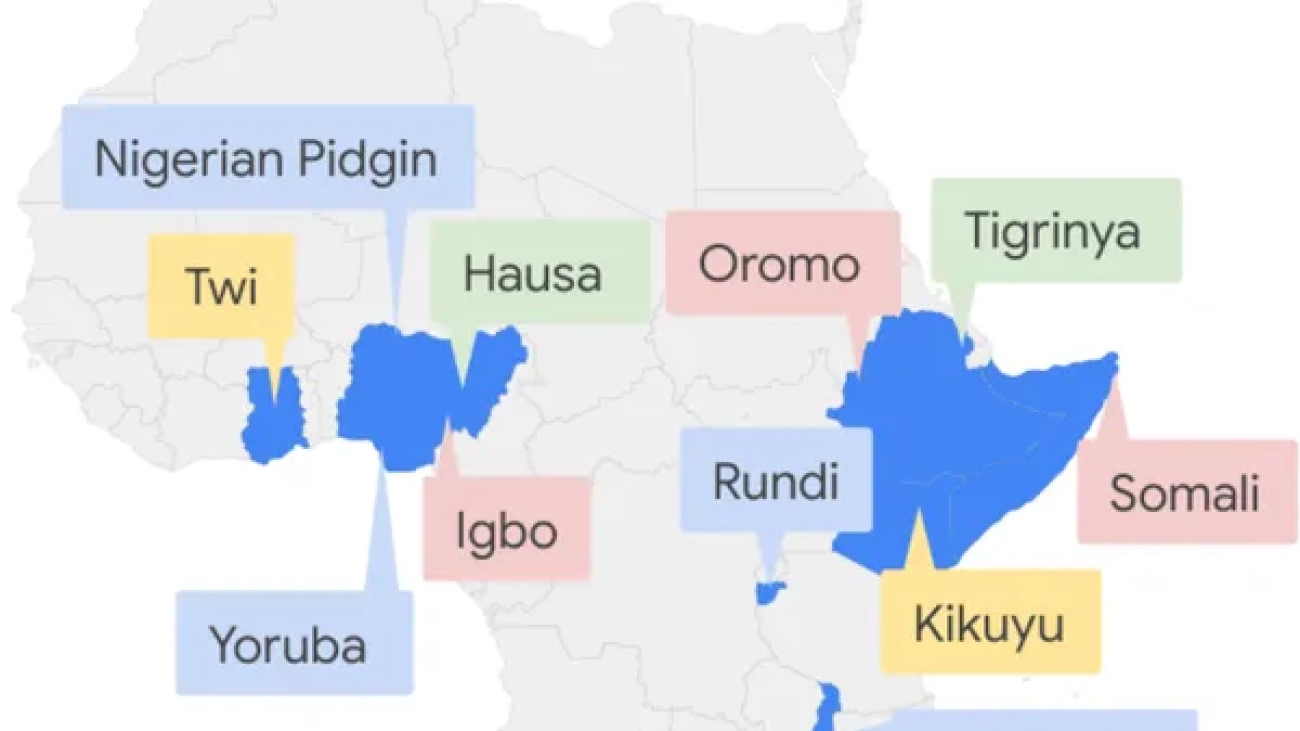
Africa’s digital decade: AI upskilling and expanding speech technology
 As Sub-Saharan Africa looks to its ‘digital decade’, President of Google EMEA Matt Brittin discusses the big opportunities and some new announcements from Google.Read More
As Sub-Saharan Africa looks to its ‘digital decade’, President of Google EMEA Matt Brittin discusses the big opportunities and some new announcements from Google.Read More
How Jacob Collier helped shape the new MusicFX DJ
 Google Labs worked with Jacob Collier to improve and simplify MusicFX DJ.Read More
Google Labs worked with Jacob Collier to improve and simplify MusicFX DJ.Read More
GOV.UK transforms its search function with help from Google Cloud
 GOV.UK, the official website of the UK government, partnered with consultancy Kin + Carta and Google Cloud to improve its user experience and transform its search engine…Read More
GOV.UK, the official website of the UK government, partnered with consultancy Kin + Carta and Google Cloud to improve its user experience and transform its search engine…Read More
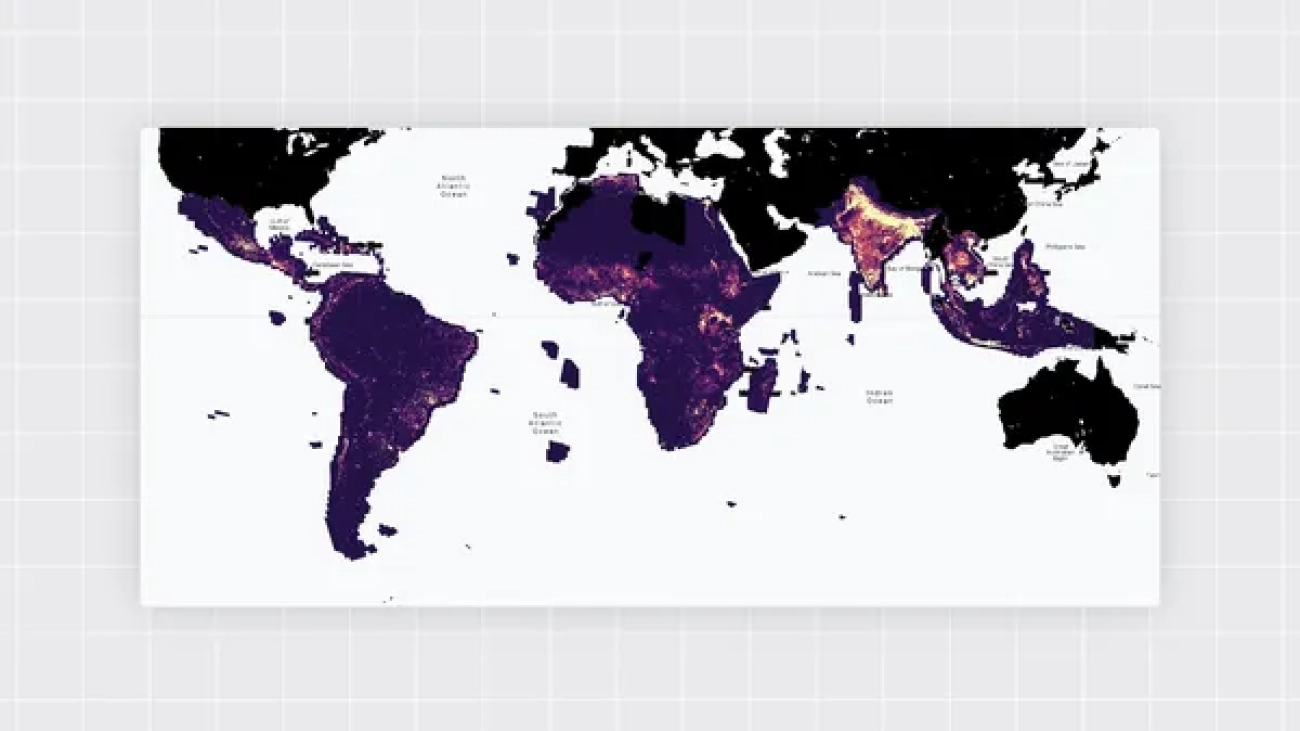
How we used AI to build our newest Open Buildings dataset
 Google’s Open Buildings 2.5D Temporal Dataset is helping to fill a major gap in data for population and density in the developing world.Read More
Google’s Open Buildings 2.5D Temporal Dataset is helping to fill a major gap in data for population and density in the developing world.Read More